Bowling Ball Nose Crusher

The classic conservation of energy demo! 

Watch The Video:

Teachable Topics:

• Conservation of Momentum
• Conservation of Energy
• Broken Cartilage

Theory:

A pendulum has potential energy due to the gravitational attraction of the earth. The equation for this potential energy (U) is

U = mgh

where m is the mass of the pendulum bob, g is the acceleration due to gravity, and h is the bob's height, measured from some arbitrary reference point.

If the pendulum is swinging, it also has kinetic energy. The equation for kinetic energy (T) is

T = (1/2)mv²

where v is the pendulum bob's velocity.

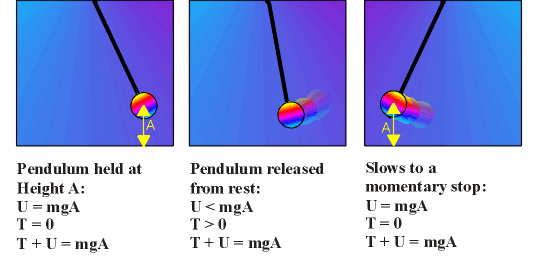
The total mechanical energy of the pendulum is E = T + U, or the sum of the potential energy and the kinetic energy. If the pendulum is left to swing freely (that is, without air resistance or friction, and without being pushed), then the total energy is conserved. It doesn't change. Potential energy can be converted to kinetic energy as the bob loses height and gains speed, or kinetic energy may be converted to potential energy as the bob swings higher and slows to a stop. Nonetheless, at every point in the pendulum's swing T + U is the same.

If the pendulum is raised to a height A and is then released from rest, at the instant of its release U = mgA and T = 0, so the total energy is

E = T + U = 0 + mgA = mgA

Since energy is conserved, T + U = mgA for the rest of the swing as well. This means that whenever the pendulum swings out and comes to rest again, it will do so at a height of A... no higher and no lower.

In a perfect situation, with no air resistance or friction on the pendulum's pivot point, this would happen. In real situations, the pendulum swings to a height lower than its starting height.

If the pendulum is again raised to a height of A, but is now given a slight push, Uagain equals mgA, but T is greater then zero. The pendulum will have enough total energy, not only to swing to a height of A, but to swing still higher. Each time the pendulum swings back again, it will come to a stop at a stop at a point higher than A.

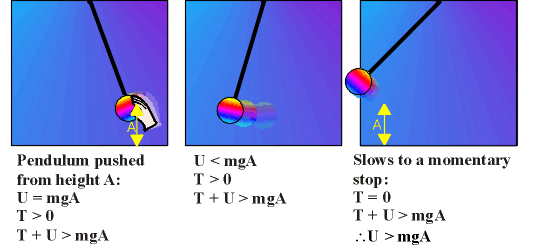
Apparatus:

• Two watermelons
• Bowling ball pendulum
• Body backboard

Procedure:

• In the first part of this demo, mount the watermelon on the wooden body backboard.
• Then take the bowling ball pendulum and push it away form the watermelon.
• The push causes the bowling ball to swing above its original height, then to swing back and crush the watermelon.
• Next, a trsuting volunteer or professor steps up with his/her back against the body backboard.
• Raise the bowling ball to the person's face, then release it without any push

SAFETY WARNINGS:

• When lifting the bowling ball up to the volunteer's nose, make sure you don't push the ball. Otherwise the volunteer may have to visit the hospital.
• The volunter must not flinch; the ball will not strike the volunteer's face unless the volunteer moves forward.
• Don't have the ball swing out over a crowd of people. Doing so might hit someone in the crowd, or a member of the crowd might interfere with the pendulum swing.